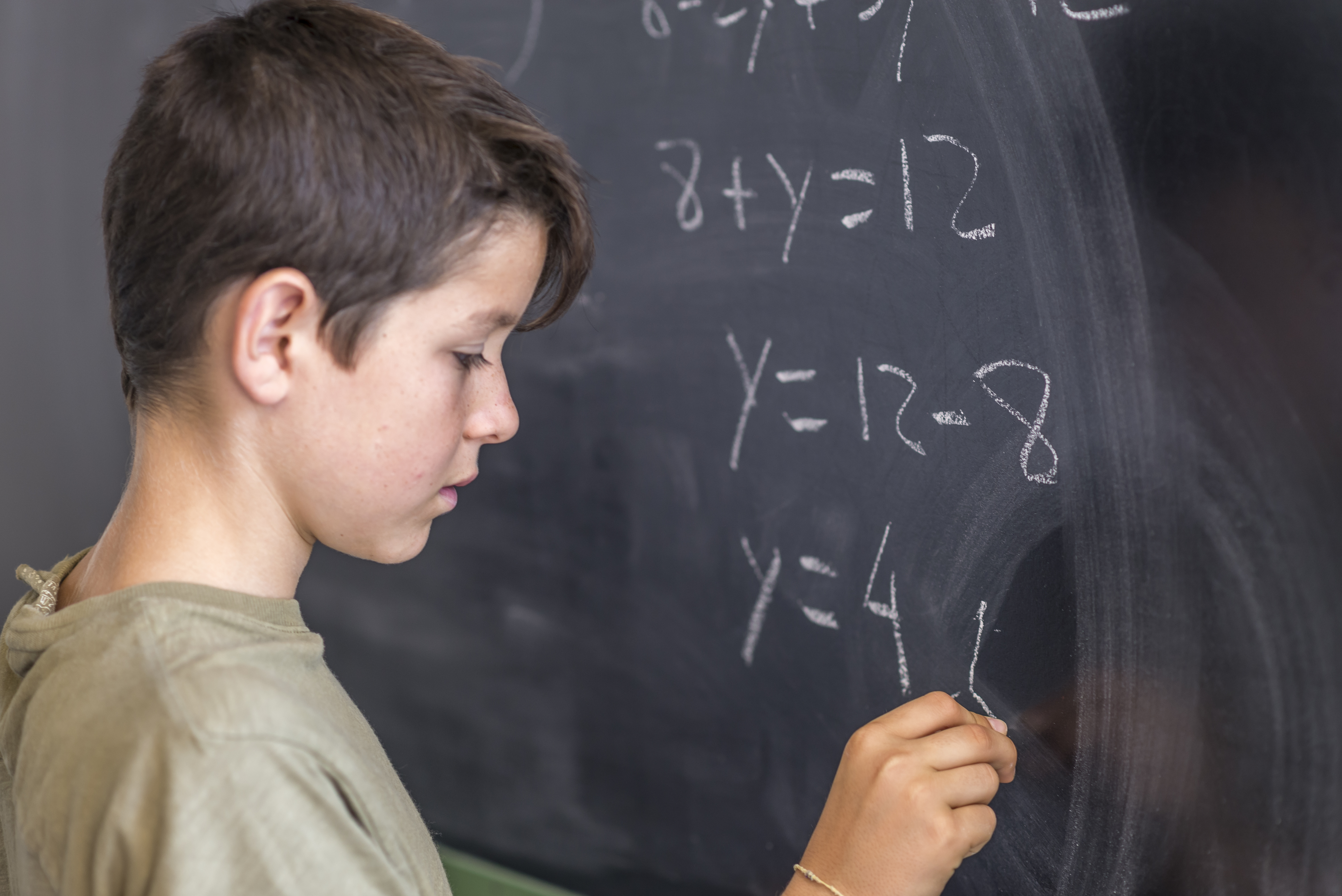Important Components of Effective Math Intervention
Students across elementary and secondary schools often experience difficulty learning math. This is problematic as students are required to meet certain benchmarks (e.g., passing algebra) in order to earn a diploma, gain admittance to college, and prepare for certain careers. If at any point in their academic career a student experiences difficulty with math, educators must provide appropriate support for the student. In the following paragraphs, I will discuss important components of effective math intervention.
First, educators should use explicit instruction to deliver high-quality and engaging instruction to students. Explicit instruction is a combination of teacher modeling, guided practice, and independent practice. During teacher modeling, the educator explains the goal of the lesson and provides step-by-step examples of the math concept or procedure. This modeling is intended to show students how to solve different types of math problems. During guided practice, the educator and student work on math problems together. During independent practice, the educator provides support as the students work independently. As modeling and practice occur, the educator should engage in a dynamic dialogue with students by asking a mix of high- and low-level questions every 30 to 60 seconds to gauge understanding, and to provide affirmative and corrective feedback.
Second, educators should focus on using appropriate math language. There are hundreds of terms that students must learn in math. Educators must provide explicit instruction on the definition of math terms, and educators must use math terms correctly. Often, educators use informal math language to help students understand math concepts (e.g., saying “bigger” or “carry”) when students need to learn the formal terms (e.g., “greater” and “regroup”). By using informal language, educators force students to learn more math language than if educators only used formal math language. Educators should also provide instruction on terms that students often confuse. For example, educators should ensure that students understand the differences between “factor” and “multiple,” or the differences among “right,” “acute,” and “obtuse.”
Third, educators should use different tools to represent math concepts and procedures, called multiple representations. Students may benefit from using three-dimensional objects (i.e., manipulatives) to touch and move around while learning new concepts. Students may also benefit from drawing pictures or using virtual manipulatives on a computer or tablet. Educators should use such tools to help students understand the abstract form of math – that is, math presented with numbers, symbols, and words.
Fourth, educators should help students build fluency. Fluency starts with fact fluency. There are 200 addition and subtraction facts and 190 multiplication and division facts, which are foundational to most following math. Educators should facilitate fluency practice daily; this fluency practice can be brief (1 to 2 minutes). There are lots of ways to practice fluency, including: flash cards, games, technology, worksheets, rolling dice, playing cards, etc. Fluency practice is best when varied from day to day, and when students practice a small set of facts. Beyond math fact fluency, students should establish efficiency with computation (i.e., adding, subtraction, multiplying, or dividing multi-digit numbers). Educators can also help establish fluency with counting objects, telling time, and counting money. In middle school, fluency can be practiced with identifying benchmark fractions or adding, subtracting, multiplying, or dividing positive and negative numbers. Building math fluency never ends!
Fifth, educators should provide effective word problem instruction to students. When students show their math knowledge, it is almost always through word problems. To create life-long problem solvers, we need to explicitly teach students how to set up and solve word problems. Educators should teach students a general attack strategy that helps students to remember to read, make a plan, solve the problem, and check their work. There are many different attack strategies, but a good strategy involves reading, planning, solving, and checking. Educators should also teach students to understand word problems at a deep level. That is, students should learn to recognize the structure of a word problem (e.g., “this problem involves a change in the amount of candies”) and then solve the problem according to a structure. There are only six structures for word problems that are used across elementary and middle school: total, difference, change, equal groups, comparison, and ratios and proportions. If students know these structures, word-problem solving across grade levels and math content is much easier.
When these five components are well-implemented and used in conjunction with one another, they should aid students experiencing difficulties in math.
Author:
Sarah Powell is an Associate Professor in the Department of Special Education at the University of Texas at Austin. After teaching kindergarten, Sarah earned her Ph.D. to conduct research related to improving math outcomes for students with learning difficulties. She currently conducts research projects related to math read-alouds in preschool, word-problem solving in elementary school, and using data to inform instruction in middle school. She can be reached at srpowell@austin.utexas.edu. You can also learn more about her work at http://www.sarahpowellphd.com/.